Stress analysis of the Francis turbine runners

(An abstract of the master thesis)

by T. Kolsek, M.Sc.

Considering the complex geometry of the water turbine components, blades, and the complex loading cases, the numerical methods only are apropriate to analyse the static and dynamic behaviour. Therefore widely used numerical finite element method (FEM) was used to determine deformation, stress and natural vibrations of the runner. The results were derived by SDRC I-DEASTM MS software, which is widely used among engineers. First, geometry was taken from appropriate drawings.
Then, the geometric model has been discretized into finite elements. Appropriate boundary conditions were chosen and loadings approximated. Finaly, results (diplacements, stresses) were obtained by linear static solver. In the following, the preparation of data, necessary computations carried out and results obtained are described.

Finite element model

The geometric model of runner was assembled of three distinct parts: crown, blade and band. The geometry of crown and band follow the drawings, whereas the surfaces of the blade were modelled by 400 points each, transfered from the test model.
ns100_13_geom_shaded.gif ns400_10_geom_ele_shaded.gif

The runner consists of 10 blades and thus shows periodic (cyclic) simmetry. The use of special boundary conditions in preparing the finite element model allows modeling of only 1/10th of the whole runner. Thus the time needed to calculate the results can greatly be reduced and/or accuracy of results improved. 1/10th of the geometric model has been discretized into Solid linear brick elements (8 nodes), which, based on general experience with FEM, are very reliable compared to 3D tetrahedrons. Care was taken to put more elements into places, where higher gradients of results were expected. Howewer, the number of elements was limited by hardware capabilities and reasonable amounts of time needed to solve the problem. At the end of discretizing, the mesh consists of 7661 elements and 9892 nodes.

Boundary conditions

In the case of static loads which repeat themselves in the same way as geometry, the deformation must reflect these periodicities and must be the same in all sectors. Therefore, the generalised displacements on the right boundary must be equal to those on the left boundary :
{uright} = {uleft}
(the components of the displacements or rotations in the cylindrical coordinate system which defines the simmetry must be equal on both boundaries. Secondly, the runner is attached to turbine shaft. The structure can therefore be considered restrained in rotation and axial displacement at the area of attachment.

Loadings

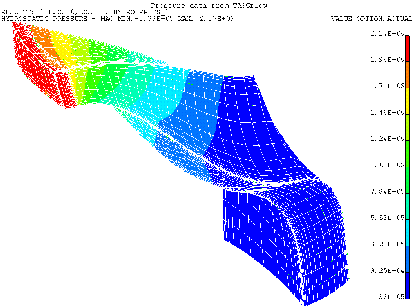
Under normal operating conditions, a Francis turbine is subjected to two sorts of static loads, namely to the centrifugal force field due to the rotational speed, and to the pressure of the water on the blades. The forces of the first type can easily be calculated and applied in finite element model, whereas the pressure distribution has been determined by the flow calculation by TASCflowTM software. The distribution is far from being uniform and analysis shows that, by taking uniform distribution into account, the results greatly differ from reality. Therefore, much effort was put to obtain the accurate pressure distribution over the blade surfaces. The data was provided by simulating the water flow through the runner and confirmed by measurements on the test site.
i
ns100_tascflow_geom_ele_shaded_napisi.gif ns100_tascflow_results.gif


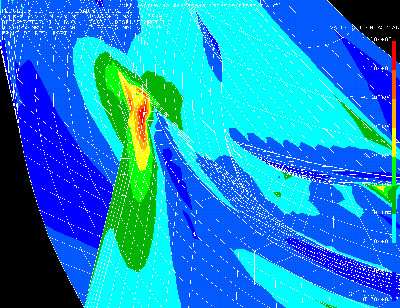
Results

The problem was solved using I-DEAS linear static solver and calculated in form of displacements, stresses and reaction forces. In general, torsional displacement of band is expressed. In the case of ns=400 runner, maximum stress 93.7 N/mm2 occurs at the blade outlet edge near the transition from blade to crown.

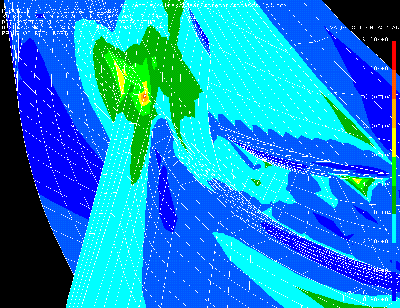
Modification

In order to lower stresses in critical area a modification of geometry has been caried out. Modified model was analyzed once again. Results show considerable fall in stress (~16%). Geometry modification is not expected to influence hydraulic characteristics of the impeller since
a) the modification takes place at the crown, where no significant energy is transfered (majority of moment is produced at greater radii)
b) beneath the impeller the air is blown into the water stream
ns400_results1.gif ALIGN = ns400_results2.gif


Conclusion

The proposed modification is easy to implement (welding at site) and expected to lower the general stress level in critical area. Analyses has shown that centrifugal force due to rotation does not contribute to stress significantly as does not slide bearing effect (due to seal clearances).